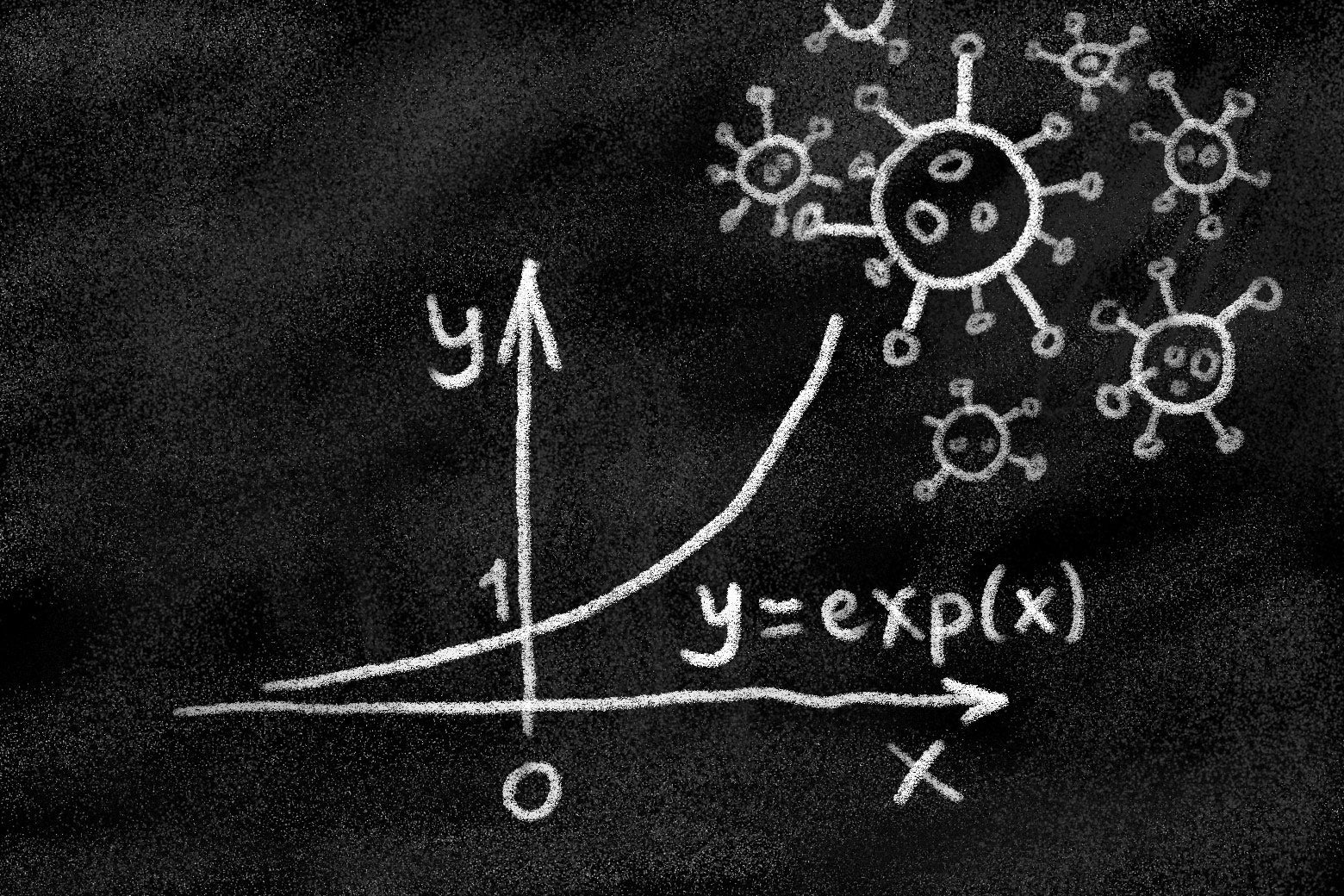Suppose you saw in the news that there was a new disease, and one person was hospitalized with it. There had been no hospitalizations before today—and yet, there had been 12,800 positive tests today for the disease, with a total of 25,500 positive tests to date, according to the news report. In short: lots of people with the disease … and basically no one suffering much for it.
I bet your first impression might be “Well, that’s not really a disease I have to worry about.”
Actually, you still may have a lot to worry about and you should draw no conclusions from this news bite, at least not without some further information. A snapshot of the current case count and hospitalizations from a disease can tell you little. You need to know a lot more about how fast the disease is spreading, and, importantly, the lag between when the cases are first detected and when those cases might result in hospitalizations.
The news bite and the numbers I provided are hypothetical (us mathematicians sometimes simplify situations for illustrative purposes). But examining them and how they can grow quickly, from a mathematical perspective, can help you understand why it feels like omicron cases snuck up so fast after the coronavirus variant was first detected and why experts worry about cases rapidly growing into an overwhelming wave of hospitalizations very quicky. (Signs point to the vaccine holding up quite well against severe disease. So if you are boosted and in good health, you can worry more about the state of the country and less about yourself.)
Cases of omicron are doubling every two or so days, according to reports from the U.K., Denmark, and now the United States. This is an example of what is called “exponential growth.” The human brain just isn’t used to understanding what happens when things are doubling constantly (or for that matter dealing with any form of exponential growth). It is accustomed to more gradual shifts—even linear growth that happens rapidly ends up being very different than exponential growth. Think about driving at a constant rate, every hour you drive, you have gone that much further. But if you don’t change your speed, the distance you cover in the next hour is exactly the same as the previous hour and so the total distance you covered increases by a constant amount. Distance covered when driving at a constant speed is a linear function! It doesn’t matter if you’ve covered hundreds of miles so far. Since this is the kind of growth we typically experience, when it comes to exponential growth, your gut feelings are going to be wrong and you need to stop and do some (elementary) math.
Exponential growth means that the amount of growth during the next time period depends on the amount of stuff there is now (by “stuff” we might mean virus, or maybe miles, if you have a very special kind of car). This is a little hard to wrap one’s head around, so let’s consider an (apocryphal, if classic) story about a chess board, recounted in a blog post on NPR. It starts with a craftsman presenting a chessboard to a king, and then:
He told the king: “Your Highness, I don’t want money for this. Or jewels. All I want is a little rice.”
“Hmm,” thought the king, who was a con man himself.
“I’ve got rice. How much rice?”
“All I want,” said the craftsman, “is for you to put a single grain of rice on the first square, two grains on the second, four on the third, eight on the fourth, and so on and so on and so on, for the full 64 squares.
“I can do that,” said the king, not thinking. And he ordered his granary to pay the man for the chessboard
How much rice would the craftsman get with this deal? Well, it is 1+ 2 + 4 +8 + 16 + 32 + 64 … doing this out for 64 times in order to fill all 64 squares of the chessboard. Which (trust me) works out to 18,446,744,073,709,551,615 grains of rice. That’s more than 18 quintillion grains of rice, which would roughly cover the planet and would be the world’s output of rice for about 1,000 years. (In this telling, the king comes out ahead by telling the craftsman he needs to count the rice himself—a task that would take many times the age of the universe.)
This is so surprising because we are accustomed to think things grow “linearly.” A version of that chess story told with linear growth might go something like: 3 grains on the first square, 6 on the second, 9 on the third, 12 on the fourth, 15 on the fifth, and so on, where the next step is gotten by adding three to the previous amount. That would result in a mere 6,240 grains of rice total which will weigh less than 5 ounces (roughly 4.62 oz actually). In general, in the linear situation, the size at any given time simply depends on what square on the chess board, so to speak, you are at—and importantly, things increase gradually, rather than on a curve that grows ever steeper. (Here is a graph that illustrates the difference between exponential and linear growth.)
Back to our disease example. In addition to cases increasing exponentially, with all versions of COVID-19 so far, hospitalizations lag behind cases by about two weeks. This makes a snapshot of cases and hospitalizations rather difficult to wrap your head around, especially when cases are high and hospitalizations are low. Two weeks doesn’t seem like so long a time, and if there’s only one hospitalization, it doesn’t feel like there could be that many more down the line. You might think: “Well, maybe I will be off by a little bit in my assumptions around the future hospitalization rate based on that news report, but how badly off can I be?” Heck, that would be my first instinct because we are all wired to think that growth is “linear.”
And now we get to the crux of the matter. In the chessboard example, rice doubles every two squares. And now, omicron appears to be doing so every two days, too. This is a very fast form of exponential growth. It means that if there is a two week (14 day) lag before a hospitalization, there will be seven doublings in the number of cases before we would see any hospitalizations from those initial cases.
Let’s plug in some numbers to show what this means in a specific situation. Suppose, for simplicity’s sake, there are 100 omicron cases today, and that cases are doubling every two days—i.e., every person seems to be infecting two new people in two days. Then, in two days, there are 200 new cases. In four days, 400 new cases. In six days, 800 new cases, etc. How many cases are there in two weeks? Well, in two weeks, we would have 12,800 new people testing positive on that day because there were seven doublings in that time.
Suppose, as may very well be true, that omicron is substantially less virulent than delta. Let’s conjecture a hospitalization rate of, say, 1 percent instead of delta’s 2.3 percent. (The rate is still being figured out.) Then, from our initial 100 cases, there would likely only have been one hospitalization two weeks after “Day Zero,” because 1 percent of a 100 is 1. But remember, we would have 12,800 positive tests for omicron on Day 14, the day that first person was hospitalized, and an astonishing 25,500 positive tests over the previous two weeks. So, on Day 15, if our conjecture that 1 percent of cases need to be hospitalized, we’d have two hospitalizations. On Day 16, there would be four. On Day 17, there would be eight. On Day 28, there would be (roughly) 128 new hospitalizations, and if our conjecture is right, by Day 28, you would have seen very close to 255 hospitalizations in total because we are assuming 1 percent of positive tests lead to a hospitalization, and we have had 25,500 positive tests so far.
There are, of course, a lot of unknowns here as to what damage omicron will do—that also depends on how we respond to it and slow it down. But, in any case, you can see how worrisome things might look within two more weeks of that sound bite that didn’t seem so bad —with all those cases doubling again and again and again.